please hurry and I will give you brainlest if your show your work
Dave has outgrown the bike he rode when he was small. So, for his twelfth birthday, his parents surprise him with a five-speed Blaze Rider 2000! Dave has never had a bike with multiple speeds before, and he is eager to learn how it works. He starts by testing the middle speed. There is a proportional relationship between the number of times Dave pedals, x, and the number of times the wheels rotate, y. What is the constant of proportionality? Write your answer as a whole number or decimal. wheel rotations per time Dave pedals

Answers
Answer:
The constant of proportionality is 2.
Step-by-step explanation:
All the y values are twice as much as the corresponding x values
Related Questions
Casho recorded the grade-level and instrument of everyone in the middle school School of Rock below.
Seventh Grade Students
Instrument # of Students
Guitar 5
Bass 10
Drums 13
Keyboard 13
Eighth Grade Students
Instrument # of Students
Guitar 9
Bass 10
Drums 10
Keyboard 4
Based on these results, express the probability that an eighth grader chosen at random will play an instrument other than drums as a fraction in simplest form.
Answers
The probability that a eighth grader chosen at random will play an instrument other than drums is a 23 / 33
What is the probability?Probability refers to a possibility that deals with the occurrence of random events.
The probability of all the events occurring need to be 1.
The formula of probability is defined as the ratio of a number of favorable outcomes to the total number of outcomes.
In this problem, we have been given Casho recorded the grade-level and instrument of everyone in the middle school School of Rock
Seventh Grade Students
5 + 10 + 13 + 13 = 41
There is a total of 41 seventh graders.
Eighth Grade Students
9 + 10 + 10 + 4 = 33
There is a total of 33 Eighth graders.
Of those, 33 - 10 = 23 play instruments that are not the drum.
Hence, the probability that an eighth grader chosen at random will play an instrument other than drums
= 23 / 33
There is a 23 / 33 probability that a eighth grader chosen at random will play an instrument other than drums.
Learn more about probability here;
https://brainly.com/question/11234923
#SPJ1
the 6% state income tax on a $42.00 salary
Answers
Answer:
75 cents
Step-by-step explanation:
A computer programming team has 17 members. (For each answer, enter an exact number.) (a) How many ways can a group of nine be chosen to work on a project? 24310 Correct: Your answer is correct. (b) Suppose nine team members are women and eight are men. (i) How many groups of nine can be chosen that contain five women and four men? 1 Incorrect: Your answer is incorrect. (ii) How many groups of nine can be chosen that contain at least one man? 9 Incorrect: Your answer is incorrect. (iii) How many groups of nine can be chosen that contain at most three women? (c) Suppose two team members refuse to work together on projects. How many groups of nine can be chosen to work on a project? (d) Suppose two team members insist on either working together or not at all on projects. How many groups of nine can be chosen to work on a project?
Answers
The number of groups that can be chosen from the team of 17 depends on
the given conditions of the selection.
The correct responses are;
(a) The number of ways of choosing groups of 9 from the 17 members are 24,310 ways.(b) (i) The number of groups of 9 containing 5 women and 4 men are 8,820 groups.(ii) The number of groups of 9 that contain at least one man is 24,309 groups.(iii) The number of groups of 9 that contain at most 3 women are 2,649 groups.(c) If two team members refuse to work together on projects, the number of groups of 9 that can be chosen are 17,875 groups.(d) If two team members must work together or not at all the number of groups of 9 that can be chosen are 1,430 groups.Reasons:
The combination of n objects taking r at a time is given as follows
\(_nC_r = \dbinom{n}{r}\)
(a) The number of ways of choosing groups of 9 from the 17 members is given as follows;
Number of ways of choosing groups of 9 = \(\dbinom{17}{9}\) = 24,310 ways.
(b) (i) Number of women = 9
Number of men = 8
Number of groups of 9 containing 5 women and 4 men = \(\dbinom{9}{5} \times \dbinom{8}{4}\)
\(\dbinom{9}{5} \times \dbinom{8}{4} = 126 \times 70 = 8,820\)
Number of groups of 9 containing 5 women and 4 men = 8,820 groups.
(ii) The number of groups that contain at least one man is \(\dbinom{17}{9} - \dbinom{9}{9}\)
\(\dbinom{17}{9} - \dbinom{9}{9} = 24,310 - 1 = 24,309\)
The number of groups that contain at least one man are 24,309 groups.
(iii) The number of groups of 9 that contain at most 3 women if given as follows;
\(\dbinom{8}{8} \times \dbinom{9}{1} + \dbinom{8}{7} \times \dbinom{9}{2} + \dbinom{8}{6} \times \dbinom{9}{3} = 2,649\)
The number of groups of 9 that contain at most 3 women are 2,649 groups.
(c) Given that two team members refuse to work together on projects, the number of groups of 9 that can be chosen are \(\dbinom{15}{9} + 2 \times \dbinom{15}{8}\)
\(\dbinom{15}{9} + 2 \times \dbinom{15}{8} = 5,005 + 2 \times 6,435 = 17,875\)
(d) Two team members must work together or not at all is \(\dbinom{15}{7} - \dbinom{15}{9}\)
\(\dbinom{15}{7} - \dbinom{15}{9} = 6,435 - 5,005 = 1,430\)
The number of groups of 9 that can be chosen two team members must work together or not at all are 1,430 groups.
Learn more here:
https://brainly.com/question/2790592
https://brainly.com/question/6949387
749/d * d/749 = 1
d=?
Answers
Answer:
D=1
Step-by-step explanation:
1. Combine multiplied terms into a single fraction
2. Cancel terms that are in both numerator and denominator
3. Divide by 1
Answer:
I honestly don't know but I think its all real numbers but not zero
Step-by-step explanation:
The manager of Tea for Us has been ordering stock based on the assumption that 51% of her customers prefer black teas. The following hypotheses are given:
H0: p = 0.51
H1: p ≠ 0.51
She sampled 158 of her customers and found that only 41% of those preferred black teas. At the 0.10 significance level, can the null hypothesis be rejected?
a. State the decision rule. (Negative answer should be indicated by a minus sign. Round the final answers to 2 decimal places.)
(Click to select)
H0
(Click to select)
H1 if z >
or z <
.
b. Compute the value of the test statistic. (Negative answer should be indicated by a minus sign. Round your answer to 2 decimal places.)
Value of the test statistic
-2.05
c. What is your decision regarding the null hypothesis?
The null hypothesis is
(Click to select)
.
Answers
a. The decision rule for this hypothesis test is: Reject H0 if the test statistic z is less than -1.645 or greater than 1.645. b. The test statistic for this hypothesis test is: -2.05.
What is hypothesis?In statistics, a hypothesis is an assumption or claim about a population parameter that can be tested by collecting and analyzing sample data.
According to given information:
a. The decision rule for this hypothesis test is:
Reject H0 if the test statistic z is less than -1.645 or greater than 1.645.
b. The sample proportion of customers who prefer black teas is:
= 0.41
The population proportion under the null hypothesis is:
p = 0.51
The sample size is:
n = 158
The test statistic for this hypothesis test is:
\(z = ( - p) / \sqrt(p * (1 - p) / n)\)
\(= (0.41 - 0.51) / \sqrt(0.51 * 0.49 / 158)\)
\(= -2.05\)
c. The test statistic z of -2.05 is less than the critical value of -1.645, so we can reject the null hypothesis H0. At the 0.10 significance level, there is enough evidence to suggest that the proportion of customers who prefer black teas is not 0.51, and the manager of Tea for Us should consider adjusting their stock orders accordingly.
To know more about hypothesis visit:
brainly.com/question/15980493
#SPJ1
PLease help, this is due tomorrow by 1 pm.

Answers
Note that the parameters of the graph a and graph b are given below.
Graph A - y=2(x-1)²
See graph attached.
Graph B - y = 1/2x² + 3
Vertex: The vertex of the function is (0, 3).
Axis of symmetry: The axis of symmetry is the vertical line passing through the vertex, which is x = 0.
Y-intercept: The y-intercept is the point where the graph intersects the y-axis. It is (0, 3).
Minimum or maximum: The coefficient of x² is positive, which means the parabola opens upwards, and therefore the function has a minimum value. The minimum value is 3.
Solutions: To find the solutions or roots of the quadratic equation, we need to set y or f(x) equal to zero and solve for x.
0 = 1/2 x² + 3
Subtracting 3 from both sides, we get:
-3 = 1/2 x²
Multiplying both sides by -2, we get:
6 = -x²
Taking the square root of both sides, we get:
x = ±√(-6)
Since the square root of a negative number is not a real number, the function has no real roots.
Minimum or maximum value: The minimum value of the function is 3.
Range: The range of the function is y ≥ 3, because the function has a minimum value of 3.
Domain: The domain of the function is all real numbers, because there are no restrictions on the values of x for which the function is defined.
Stretch/Shrink/Standard: The coefficient of x^2 is positive and less than 1, which means that the graph of the function is narrower than the graph of y = x². This is an example of a standard quadratic function that has been vertically compressed by a factor of 1/2.
See graph attached.
Learn more about graphs at:
https://brainly.com/question/17267403
#SPJ1


Insert < >, or > in the appropriate space to make a true statement. | -8] __ -1
Answers
-8 < -1
hope this helps :)
What is the slope-intercept equation
for the following line?
Enter the correct
symbol,+ or -
y = [?][]+[]
![What is the slope-intercept equationfor the following line?Enter the correctsymbol,+ or -y = [?][]+[]](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/gTE67iSH6LNeMEJw6hRWYBV8yCvQXxe2.png)
Answers
Answer:
y = - x + 1
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (0, 1) and (x₂, y₂ ) = (4, - 3) ← 2 points on the line
m = \(\frac{-3-1}{4-0}\) = \(\frac{-4}{4}\) = - 1
the line crosses the y- axis at (0, 1 ) ⇒ c = 1
y = - x + 1 ← equation of line
Solve the following system of equations with the substitution method:
y=3/5x-15
y=-3/4x+12
Answers
Answer:
x = 20, y = -3
Step-by-step explanation:
Set both equations equal to each other
\(\displaystyle y=\frac{3}{5}x-15\\\\y=-\frac{3}{4}x+12\\\\\\\\\frac{3}{5}x-15=-\frac{3}{4}x+12\\\\\frac{12}{20}x-15=-\frac{15}{20}x+12\\\\\frac{27}{20}x-15=12\\\\\frac{27}{20}x=27\\\\x=20\\\\y=\frac{3}{5}x-15\\\\y=\frac{3}{5}(20)-15\\\\y=\frac{60}{5}-15\\\\y=12-15\\\\y=-3\)
Please help me with the answer ASAP

Answers
Answer:
y = 4x + 1
Step-by-step explanation:
y = mx + b
b = y-intercept
From the graph we see that b = 1
m = slope
Start at (0, 1). Go up 8 units. Rise = 8. Go right 2 units. Run = 2.
slope = rise/run = 8/2 = 4
y = 4x + 1
A box of colored crayons contains 11 distinct colors. In how many ways can 2 colors be chosen, assuming that the order of the colors chosen doesn’t matter? (If necessary consult a list of formulas.)
Answers
Answer:
55
Step-by-step explanation:
The first crayon is chosen out of 11,so there are 11 choices
11
The second crayon is chosen out of 10 crayons left so there are 10 choices
10
11*10 = 110
But we do not care about the order, so we divide by 2 since there are 2 places
110/2 =55
Answer:
55
Step-by-step explanation:
Equation is a = n * (n-1)
11 * 10 = 110
Order doesn't matter, and two colors, so divide by 2.
110/2 = 55
simplify without using a calculator :
[log_{x}(64) + log_{x}(4) - log_{x}(8)] ÷[log_{x}(1024)]
Answers
Since log(ab) = log(a) + log(b) and log(a/b) = log(a) - log(b) for any logarithm base, we have
\(\dfrac{\log_x(64) + \log_x(4) - \log_x(8)}{\log_x(1024)} = \dfrac{\log_x\left(\dfrac{64\times4}8\right)}{\log_x(1024)} = \dfrac{\log_x(32)}{\log_x(1024)}\)
Then using the change-of-base identity, we have
\(\dfrac{\log_x(32)}{\log_x(1024)} = \log_{1024}(32)\)
But we also know that 2¹⁰ = 1024 and 2⁵ = 32, so we can back up slightly and instead write
\(\dfrac{\log_x(32)}{\log_x(1024)} = \dfrac{\log_x(2^5)}{\log_x(2^{10})} = \dfrac{5\log_x(2)}{10\log_x(2)}\)
Then the logarithms cancel and you're left with 5/10 = 1/2.
can someone pleaseeeeee help me with this??!!

Answers
Answer:
49
Step-by-step explanation:
Answer:
The answer is 49 :)
Step-by-step explanation:
Each week in math class, Haley takes a quiz with 5 questions. She earns 2 points for each correct answer and loses 1 point for each incorrect answer. If she answers 4 questions correctly and 1 question incorrectly on each quiz, how many points will she earn after 6 weeks?
Answers
Answer:
42
Step-by-step explanation:
4x2x6=48
48-6=42
Answer:
42
Step-by-step explanation
8 - 1 * 6 = 42
A raindrop fallsat a rate of 4/50mile in 1/20hour. At this rate, how far will the raindroptravelin 1 hour?(60 minutes)
Answers
Answer:
Step-by-step explanation:
1/20 hour = 5 minutes
(We can now call 1/20 of an hour 5 minutes)
If it falls 4/50 of a mile in 5 minutes (1/20 of an hour),
we do 4/50 * 12 because there are 12 5-minute intervals in an hour/
4/50 * 12 = 48/50 = 24/25 of a mile
Therefore, the raindrop will travel 24/25 of a mile in an hour.
The distance of the raindrop in an hour will be 24/25 miles.
What is speed?The speed is defined as the ratio of the time distance traveled by the body to the time taken by the body to cover the distance. Speed is a scalar quantity and is described by its magnitude only.
Given that A raindrop falls at a rate of 4/50 mile in 1/20 hour. The distance in an hour will be calculated as:-
If it falls 4/50 of a mile in 5 minutes ( 1/20 of an hour ),
Distance = 4/50 x 12
Distance = 4/50 x 12
Distance = 48/50
Distance = 24/25 of a mile
Therefore, the distance of the raindrop in an hour will be 24/25 miles.
To know more about Speed follow
https://brainly.com/question/6504879
#SPJ2
Estimate a 15% tip on a dinner bill of $79.24 by first rounding the bill amount to the nearest ten dollars.
Answers
Hope it helps!
Determine the equation of the tangent line in both cases
1. x^2/x+2 at (2,1)
2. x^3+2y^2=10y at (2,1)
Answers
Differentiate the function/equation with respect to x and solve for the derivative, dy/dx. The value of dy/dx at the given point is the slope of the tangent line to the curve at that point. Then use the point-slope formula to get the equation of the tangent.
1.
\(y = \dfrac{x^2}{x+2} \implies \dfrac{dy}{dx} = \dfrac{2x\times(x+2) - x\times1}{(x+2)^2} = \dfrac{x(x+4)}{(x+2)^2}\)
When x = 2, the derivative is
\(\dfrac{dy}{dx}\bigg|_{x=2} = \dfrac{2(2+4)}{(2+2)^2} = \dfrac34\)
Then the equation of the tangent line at (2, 1) is
\(y - 1 = \dfrac34 (x - 2) \implies \boxed{y = \dfrac{3x}4 - \dfrac12}\)
2.
\(x^3 + 2y^2 = 10y \implies 3x^2 + 4y \dfrac{dy}{dx} = 10 \dfrac{dy}{dx} \implies \dfrac{dy}{dx} = \dfrac{3x^2}{10-4y}\)
When x = 2 and y = 1, the derivative is
\(\dfrac{dy}{dx}\bigg|_{(x,y)=(2,1)} = \dfrac{3\times2^2}{10-4\times1} = 2\)
Then the tangent at (2, 1) has equation
\(y - 1 = 2 (x - 2) \implies \boxed{y = 2x - 3}\)
The graph of function f is shown. Function g is represented by the table. x -1 0 1 2 3 4 g(x) 24 6 0 -2 Which statement correctly compares the two functions? A. They have different end behavior as x approaches -∞ and different end behavior as x approaches ∞. B. They have the same end behavior as x approaches -∞ but different end behavior as x approaches ∞. C. They have the same end behavior as x approaches -∞ and the same end behavior as x approaches ∞. D. They have different end behavior as x approaches -∞ but the same end behavior as x approaches ∞.
Answers
The answer choice which correctly draws a comparison between the end behaviors of the functions is; Choice C; They have the same end behavior as x approaches -∞ and same end behavior as x approaches ∞.
As given in the task content; the graph of the exponential function passes through (-4, 9), (0, 1), (1, 0), and (5, -2).
It therefore follows that as; x reduces (approaches -∞), the y value increases.
And, as x -increases (approaches +∞), the y-value decreases.
For the table, the values can be written as coordinates as follows; (-1,2), (0,4), (1,6), (2, 0), (3,-2).
Consequently, as x reduces (approaches -∞), the y- values decrease.
And, as x increases (approaches +∞), the y-values decrease.
Ultimately, the two functions in discuss can be concluded to have; Choice C.
Read more on end behaviour of functions;
brainly.com/question/1365136
#SPJ1
16
Select the correct answer.
Which of the following graphs shows the solution set for the inequality below?
Ix+21+7>10
OA HH
OB. H
5
1 0 1 2 3
0 1
2 3 4
10
5 6

Answers
The solution set includes all real numbers less than -5, and all real numbers greater than 1, but does not include -5 or 1 themselves.
Option D is the correct answer.
We have,
We need to isolate the absolute value |x + 2| on one side of the inequality.
We subtract 7 from both sides.
|x + 2| > 3
Next, we can split this inequality into two separate inequalities, one for when the expression inside the absolute value is positive, and one for when it is negative:
x + 2 > 3
or
- (x + 2) > 3
Solving for x in the first inequality.
x > 1
Solving for x in the second inequality.
-x - 2 > 3
Adding 2 to both sides and multiplying by -1.
x < -5
So the solution set for the inequality |x + 2| + 7 > 10 is the set of all x-values that satisfy either x > 1 or x < -5.
Using interval notation.
(-∞, -5) U (1, ∞)
Thus,
The solution set includes all real numbers less than -5, and all real numbers greater than 1, but does not include -5 or 1 themselves.
Learn more about inequalities here:
https://brainly.com/question/20383699
#SPJ1
Solve the inequality for w.
w+7<20
Simplify your answer as much as possible.
0
Answers
Answer:
w<13
Step-by-step explanation:
Works identically to a normal single-variable equation.
Subtract 7 on both sides in order to isolate w--->w+7-7<20-7
The answer (which cannot be simplified any further) is w<13.
Answer:
w < 1`3
Step-by-step explanation:
Isolate the variable w on one side of the inequality sign.
w+7<20
w<20 - 7
w<13.
IM IN A HURRY PLEASE HELP ME QUESTION IS DOWN BELOW WORTH 15 POINTS each

Answers
Applying the congruent chords theorem, the value of x is: 7.
Length of segment LP is: 6 units.
What is a Chord in Geometry?In geometry, a chord is defined as the line segment that joins two points on the circumference of a circle.
What is the Congruent Chords Theorem?In a circle, if two chords are congruent, then they are equidistant from the center of a circle, according to the congruent chords theorem.
In the circle given, chords JK = LM = 12 units. This means both chords are congruent, therefore, they are equidistant from the center of the circle S.
According to the congruent chords theorem, NS = PS, thus:
8 = 2x - 6
8 + 6 = 2x
14 = 2x
Divide both sides by 2
14/2 = 2x/2
7 = x
x = 7
LP = 1/2(12)
LP = 6 units.
In summary, applying the congruent chords theorem, the value of x is: 7.
Length of segment LP is: 6 units.
Learn more about the congruent chords theorem on:
https://brainly.com/question/7511582
#SPJ1
Please please fast I need it fast

Answers
Answer:
0
Step-by-step explanation:
Eliminate the opposite
Answer:
Its same as
a-a
=0
Have a great day!
Hold on hold on hold on hold on hold on oh my God if seven is an element in the domain of F parentheses X parentheses equals 6X -19 over five what is the corresponding element in the range
Answers
The corresponding element in the range of the function F when 7 is in the domain is 4.6.
The expression for the given function F following as:
F(x) = 6(x) - 19 / 5
The corresponding element in the range of the function F is the output of the function when 7 is input into the function.
To find this output, we can substitute 7 for X in the expression for the function F:
F(7) = 6(7) - 19 / 5
F(7) = 42 - 19 / 5
F(7) = 23 / 5
F(7) = 4.6
Thus, when 7 is in the domain, the equivalent element in the range of the function F is 4.6.
Learn more about the domain and the range here:
brainly.com/question/21027387
#SPJ1
Find the radius of a circle that has an area of 121 pi ft2.
Answers
Answer: 11
Step-by-step explanation:
area = 121 pi = pi r^2 Divide both sides by pi
121 = r^2
r= 11
1482 : ( 147 + 10 x X) = 6
Answers
Please click above answer

Find the area of the semicircle. Round your answer to the nearest hundredth. YEAH IVE BEEN STUCK ON THIS help please I need the steps if possible:(
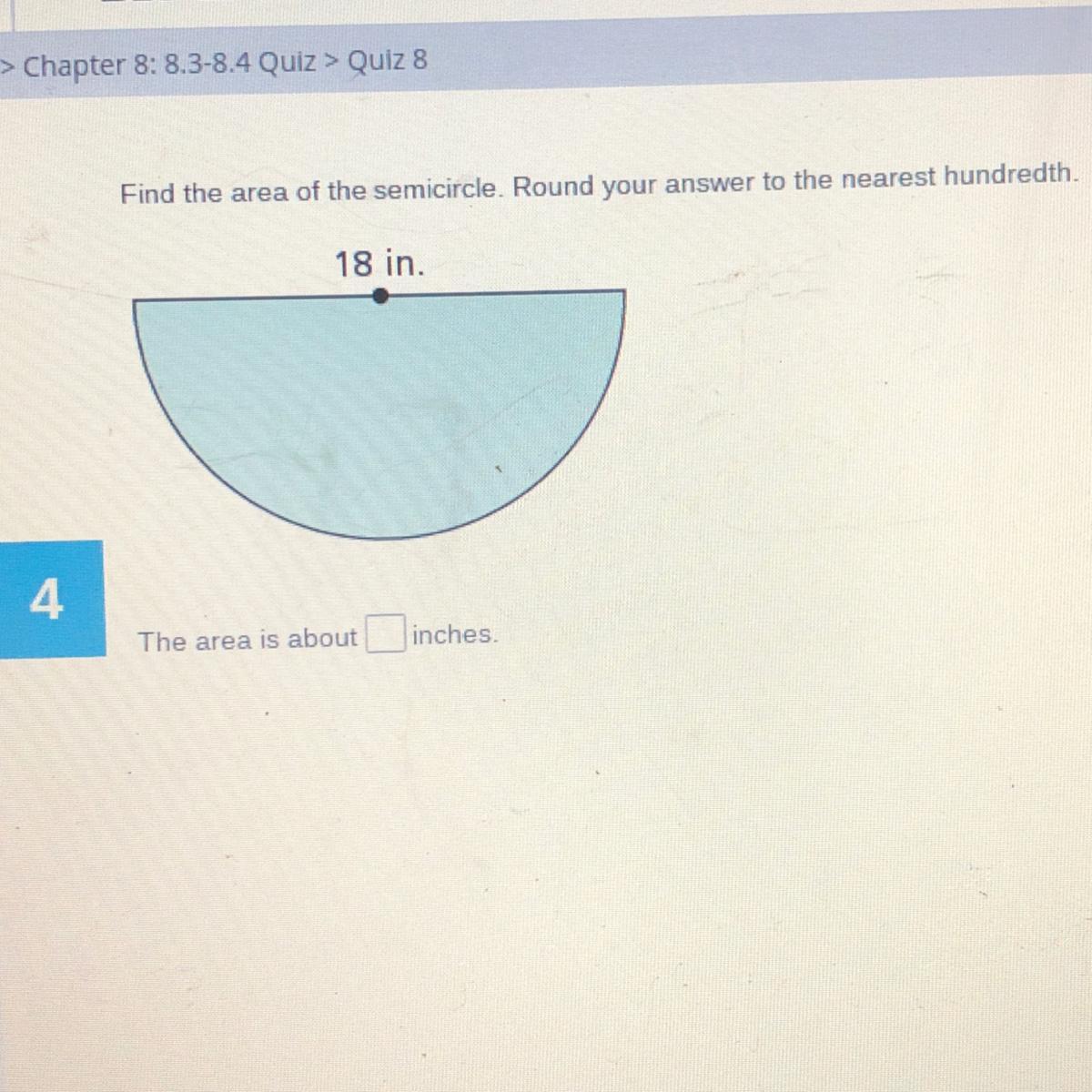
Answers
Answer:
your answer will be 127.17 sq in
Step-by-step explanation:
hope it helps you
have a wonderful day!!!

Answer:
=127.23
Step-by-step explanation:
we can start of by finding the area of a CIRCLE
18/2=9
9=radius
area of circle= pi*r squared
9 squared=81*pi=254.4690
Now we can half the area of the circle to find out the area of the semi circle as a semi circle is just half a circle therefore half the area of a circle
254.469/2=127.2345
127.2345=127.23
=127.23
Hope this helped
please answer correctly thanks
mamaya napo pasahan ng 10 am pasagot po please

Answers
wag po lagi umasa sa brainly
what is the sum of 5/6+2/3
Answers
Step-by-step explanation:
\( \frac{5}{6} + \frac{2}{3} \)
\( \frac{5}{6} + \frac{2 \times 2}{3 \times 2} \)
\( \frac{5}{6} + \frac{4}{6} \)
\( = \frac{5 + 4}{6} \)
\( = \frac{9}{6} \)
\( = \frac{3}{2} \)
\( = 1 \frac{1}{2} \)
Answer:
1 1/6 (read one and one-sixth) or 11/6
Step-by-step explanation:
2/3=4/6
5/6+4/6=11/6 or 1 1/6
Suppose MarketOne is a marketing company that has small businesses for clients. MarketOne charges an upfront cost of $350 for new clients and an additional $195 per month to run and maintain the client's website. A linear equation could be used to relate the total cost, in dollars, of MarketOne's services and the number of months that the client's website is running. Which solution is viable for this situation? For full points, write the equation and show your work.
Answers
C = 195m + 350
where the constant term of 350 represents the upfront cost and the coefficient of 195 represents the monthly cost.
To check if this equation is viable for the situation, we can use some reasonable values for the number of months and see if the resulting costs make sense.
For example, if the client's website is running for 6 months, the total cost would be:
C = 195(6) + 350
C = 1,170 + 350
C = 1,520
This means that the client would pay $1,520 in total for 6 months of service from MarketOne.
Similarly, if the client's website is running for 12 months, the total cost would be:
C = 195(12) + 350
C = 2,340 + 350
C = 2,690
This means that the client would pay $2,690 in total for 12 months of service from MarketOne.
Based on these calculations, we can see that the linear equation is viable for the situation. The equation allows us to calculate the total cost of MarketOne's services based on the number of months the client's website is running, which is a useful tool for budgeting and planning.
State the domain and range for each graph and then tell if the graph is a function(write yes or no)
Answers
For the point 1)
- The domain will be: (note that this is not an interval, it is a set of two points)
\(\mleft\lbrace-3,2\mright\rbrace\)-The range is the set R of all real numbers (since the line extends to infinite)
-The first graph is NOT a function
For the point 2)
-The domain will be the interval
\((-5,5\rbrack\)-The range is the interval:
\(\lbrack-2,2\rbrack\)-The second graph is a function.