In December 2018, the average price of regular unleaded gasoline excluding taxes in the United States was $3.06 per gallon
Assume that the standard deviation price per gallon is $0.05 per gallon and use Chebyshev's Inequality to answer the following
(a) What minimum percentage of gasoline stations had prices within 2 standard deviations of the mean?
(b) What minimum percentage of gasoline stations had prices within 2.5 standard deviations of the mean?
(c) What is the minimum percentage of gasoline stations that had prices between $2.91 and $3.21?
Answers
Using Chebyshev's Theorem, it is found that:
a) The minimum percentage is 75%.
b) The minimum percentage is 84%.
c) The minimum percentage is 89%.
By Chebyshev's Theorem, the minimum percentage of measures within k standard deviations of the mean is:
\(P = 100\left(1 - \frac{1}{k^{2}}\right)\)
Item a:
Within 2 standard deviations, hence k = 2, and:
\(P = 100\left(1 - \frac{1}{2^{2}}\right) = 100\left(\frac{3}{4}\right) = 75\)
The minimum percentage is 75%.
Item b:
2.5 standard deviations, hence k = 2.5, and:
\(P = 100\left(1 - \frac{1}{2.5^{2}}\right) = 84\)
The minimum percentage is 84%.
Item c:
3 standard deviations, hence k = 3, and:
\(P = 100\left(1 - \frac{1}{3^{2}}\right) = 89\)
The minimum percentage is 89%.
A similar problem is given at https://brainly.com/question/15050238
Related Questions
find the domain of f(x) = 3x + 5
Answers
Answer:
All real numbers
Step-by-step explanation:
All real numbers: f(x) is defined for all values of x. There are no discontinuities or places where f(x) is undefined. f(x) is just a straight line going to infinity.
Answer:
(-infinity, infinity), or all real numbers
Step-by-step explanation:
If you were to look at it graphically, it would be a linear function with a slope of 3 and shifted up 5 units. (i attached a screenshot of what the graph would look like at the bottom :)
since there are no domain restrictions, you're not really going to have any issues or gaps which is when you might need to do something like
(-infinity,4] u(5,inifinity) --> since this isn't the case though, you're totally free to just write all real numbers, because all real numbers in the x-plane (as that is what domain looks at) are included.
When all else fails, (at least for homework), desmos is great for graphing and looking at everything visually
Hope that helps! lmk if you have any questions :)

Acylinder has a volume of 400 cubic feet. If the height of the cylinder is 25 feet, what is the radius of the cylinder? Use 3.14 for me and round to the nearest hundredth
adius hype your answer...
1
Answers
The radius of the cylinder is 2.25 feet.
To find the radius of the cylinder, we can use the formula for the volume of a cylinder:
V = πr²h
Given that the volume V is 400 cubic feet and the height h is 25 feet, we can substitute these values into the formula and solve for the radius r.
400 = 3.14 r² x 25
Dividing both sides of the equation by (3.14 * 25) to isolate r^2, we have:
r² = 400 / (3.14 x 25)
r² ≈ 5.08
Taking the square root of both sides to solve for r, we get:
r ≈ √5.08
r ≈ 2.25
Therefore, the radius of the cylinder is 2.25 feet.
Learn more about Volume here:
https://brainly.com/question/28058531
#SPJ1
Tom and John are engaged in buying and selling certain products A and B. Tom BUYS 5 of product A but
SELLS twice as much of product B. John on the other hand SELLS three times what Tom BOUGHT of
product A and BUYS 13 of product B. At the end of the business day, John banks Ksh 110,000/- while
Tom banks Ksh 230,000.
Under the assumption that the sale prices for product A and B are the same for the two men, and the costs prices for the products A and B are also the same for the two men, obtain the following:
The price for product A which was determined as Ksh 42,727.27 and the price for product B as 44,363.63
Question: If there was a mark up of 25% on the cost price and a discount of 15% on the sale price, how
much would each of the partners have banked at the end of the business day?
Answers
Tom would bank Ksh 277,272.73 and John would bank Ksh 693,939.39 at the end of the business day with 25% markup.
What is markup?The markup is the discrepancy between a product's selling price and its cost price. Adding a particular percentage to the cost price to arrive at the selling price of a product is a frequent practise in business. Depending on the sector, the level of competition, and other elements, the markup % may change.
Since it influences a product's or service's profitability, markup is significant in business. If the markup is too low, the company could not have a sufficient profit to pay its bills and continue operating. The product could not be competitive with other similar items on the market if the markup is too high.
Let us suppose the cost price and sales price = x.
Thus,
For Tom:
Cost of 5 A + Cost of 2B = 5x + 2x = 7x
Sale of 10 B = 10(2x) = 20x
Here,
Profit = Sale - Cost = 20x - 7x = 13x
For the given value of profit we have:
Tom's profit = Ksh 230,000
13x = 230,000
x = 17,692.31
Simillarly for Jhon:
or John:
Sale of 3 A = 3(5x) = 15x
Cost of 13 B = 13x
Profit = Sale - Cost = 13x - 15x = -2x
John's profit = Ksh 110,000
-2x = 110,000
x = -55,000
Now, the markup is 25%,:
New cost price for John = 17,692.31
New sale price for John = 17,692.31 + 0.25(17,692.31) = 22,115.39
Actual sale price for John = 22,115.39 - 0.15(22,115.39) = 18,798.08
The new profit is thus:
New cost price for John = 17,692.31
New sale price for John = 17,692.31 + 0.25(17,692.31) = 22,115.39
Actual sale price for John = 22,115.39 - 0.15(22,115.39) = 18,798.08
Hence, Tom would bank Ksh 277,272.73 and John would bank Ksh 693,939.39 at the end of the business day.
Learn more about markup here:
https://brainly.com/question/11999225
#SPJ1
Find the values of a,b and c if
ax^2 -2ax -bx -2b +c=x^2 + 2x +1
Answers
Answer:
Step-by-step explanation:
In accordance with the Remainder theorem, the given part means that the given polynomial has the roots of 1, -2 and 2.
So we write these three equations expressing this fact
2A*1^4 - B*1^3 - C*1 - 16 = 0
2A*(-2)^4 - B*(-2)^3 - C*(-2) - 16 = 0
2A*2^4 - B*2^3 - C*2 - 16 = 0
or
2A - B - C = 16 (1)
32A + 8B + 2C = 16 (2)
32A - 8B - 2C = 16 (3)
Adding equations (2) and (3), you get
64A = 32, which implies A = 32%2F64 = 0.5.
Then from (1) and (2), substituting A = 0.5 there, you get
B + C = -15 (4)
8B + 2C = 0 (5)
Expressing B = -15 - C from (4) and substituting it to (5), you get
8*(-15 -C) + 2C = 0, or
-120 - 8C + 2C = 0
- 6C = 120
C = - 20.
Then from equation (4), B = -15 - (-20) = -15 + 20 = 5.
ANSWER. A = 0.5; B = 5, C = -20.
We have three factors: (x-1),(x+2) and (x-2)
However, we have a quartic polynomial, since the degree (largest exponent) is 4
So we need a fourth factor. Let's call that x-p, for some real number p.
Let's expand out the following
(x-1)(x+2)(x-2)(x-p)
(x+2)(x-2)(x-1)(x-p)
(x^2-4)(x^2-px-x+p)
x^2(x^2-px-x+p)-4(x^2-px-x+p)
x^4-px^3-x^3+px^2-4x^2+4px+4x-4p
x^4+(-px^3-x^3)+(px^2-4x^2)+(4px+4x)-4p
x^4+(-p-1)x^3+(p-4)x^2+(4p+4)x-4p
Compare that result to 2Ax^4-Bx^3-Cx-16
Match up the corresponding terms, and we have the following equations
2Ax^4 = 1x^4 which leads to 2A = 1 and solves to A = 1/2 = 0.5
(-p-1)x^3 = -Bx^3 which leads -p-1 = -B and solves to B = p+1
(4p+4)x = -Cx which leads to 4p+4 = -C and solves to C = -4p-4
-4p = -16 which solves to p = 4
Use that value of p to find the other coefficients
B = p+1 = 4+1 = 5
C = -4p-4 = -4*4-4 = -20
The polynomial 2Ax^4-Bx^3-Cx-16 turns into x^4-5x^3+24x-16 after we plug in those A,B, and C values.
Answers:
A = 1/2 = 0.5
B = 5
C = -20
Find answers here!
https://www.algebra.com/algebra/homework/Polynomials-and-rational-expressions/Polynomials-and-rational-expressions.faq.question.1170859.html
Find x, y, and z.
10.
Z
Х
5
7
Answers
Answer: =10
Step-by-step explanation:
consider a random experiment of tossing a biased coin three times. we assume that the tosses are independent of each other and the probability of a head is 0.4. let x be the random variable whose value represents the number of heads obtained.
Answers
The probability of getting 1 head out of the 3 tosses is 0.432.
In this random experiment, we are tossing a biased coin three times, with the probability of getting a head in a single toss being 0.4.
We assume that the tosses are independent of each other.
Let X be the random variable that represents the number of heads obtained.
To determine the probability distribution of X, we can use the binomial distribution formula, which is:
P(X = k) = (n choose k) * \(p^k * (1 - p)^(n - k)\)
where
n is the number of trials,
k is the number of successes,
p is the probability of success, and
(n choose k) is the binomial coefficient, which represents the number of ways to choose k objects from a set of n objects.
In this case, we have n = 3 and p = 0.4. So, the probability of getting exactly k heads is:
P(X = 0) = \((3\: choose \:0) * 0.4^0 * 0.6^3\) = 0.216
P(X = 1) = \((3\: choose\: 1) * 0.4^1 * 0.6^2\) = 0.432
P(X = 2) = \((3 \:choose \:2) * 0.4^2 * 0.6^1\)= 0.288
P(X = 3) =\((3 \:choose \:3) * 0.4^3 * 0.6^0\) = 0.064
Therefore, the probability distribution of X is:
X 0 1 2 3
P(X) 0.216 0.432 0.288 0.064
This distribution shows that the most likely outcome is to get 1 head out of the 3 tosses, with a probability of 0.432.
For similar questions on probability,
https://brainly.com/question/24756209
#SPJ4
NO LINKS!! URGENT HELP PLEASE!!
Using the information given in each diagram below, decide if any triangles are congruent, similar but not congruent, or similar. If you claim the triangles are congruent or similar, create a flowchart justifying your answer. Part 1
Please help me with #22 and 24

Answers
Answer:
22. Congruent traingle
24. Similar traingle
We need to compare their corresponding sides and angles to determine if the two triangles are congruent or similar.
Attached flowchart to help you determine if two triangles are congruent or similar: See Attachment:
Properties of the similar triangle:
Corresponding angles are congruent: The corresponding angles of similar triangles are congruent. This means that if we label the corresponding angles of two similar triangles as A, B, and C and A', B', and C', respectively, then angle A is congruent to angle A', angle B is congruent to angle B', and angle C is congruent to angle C'.Corresponding sides are proportional: The corresponding sides of similar triangles are proportional. This means that if we label the corresponding sides of two similar triangles as a, b, and c and a', b', and c', respectively, then a:b::a':b' and b:c::b':c'.Ratios of corresponding sides are equal: The ratios of the corresponding sides of similar triangles are equal. This means that a/b = a'/b' and b/c = b'/c'.Perimeters and areas are proportional: The perimeters of similar triangles are proportional to the ratios of their corresponding sides, and the areas are proportional to the ratios of their corresponding sides squared. For example, if the ratio of the corresponding sides of two similar triangles is 2:3, then the ratio of their perimeters is also 2:3, and the ratio of their areas is 4:9.Height and base are proportional: The height of similar triangles is proportional to the ratios of their corresponding sides. This means that if two similar triangles have heights h and h', respectively, and corresponding sides a and a', then h/h' = a/a'. Similarly, if the triangles have bases b and b', then the ratio of their heights is also b/b'.Properties of the Congruent Triangle:
Corresponding sides and angles are congruent: In congruent triangles, corresponding sides and angles are congruent. This means that if we label the corresponding angles of two congruent triangles as A, B, and C and A', B', and C', respectively, then angle A is congruent to angle A', angle B is congruent to angle B', angle C is congruent to angle C', and side AB is congruent to side A'B', side AC is congruent to side A'C', and side BC is congruent to side B'C'.Congruent triangles have equal perimeters: Congruent triangles have the same size, so their perimeters are equal.Congruent triangles have equal areas: Congruent triangles have the same size, so their areas are equal.The sum of interior angles is always 180 degrees: The sum of the three interior angles of a triangle is always 180 degrees, regardless of whether the triangle is congruent or similar.The altitude, median, and angle bisector of a triangle are also congruent: In congruent triangles, the altitude, median, and angle bisector of one triangle are congruent to the corresponding altitude, median, and angle bisector of the other triangle.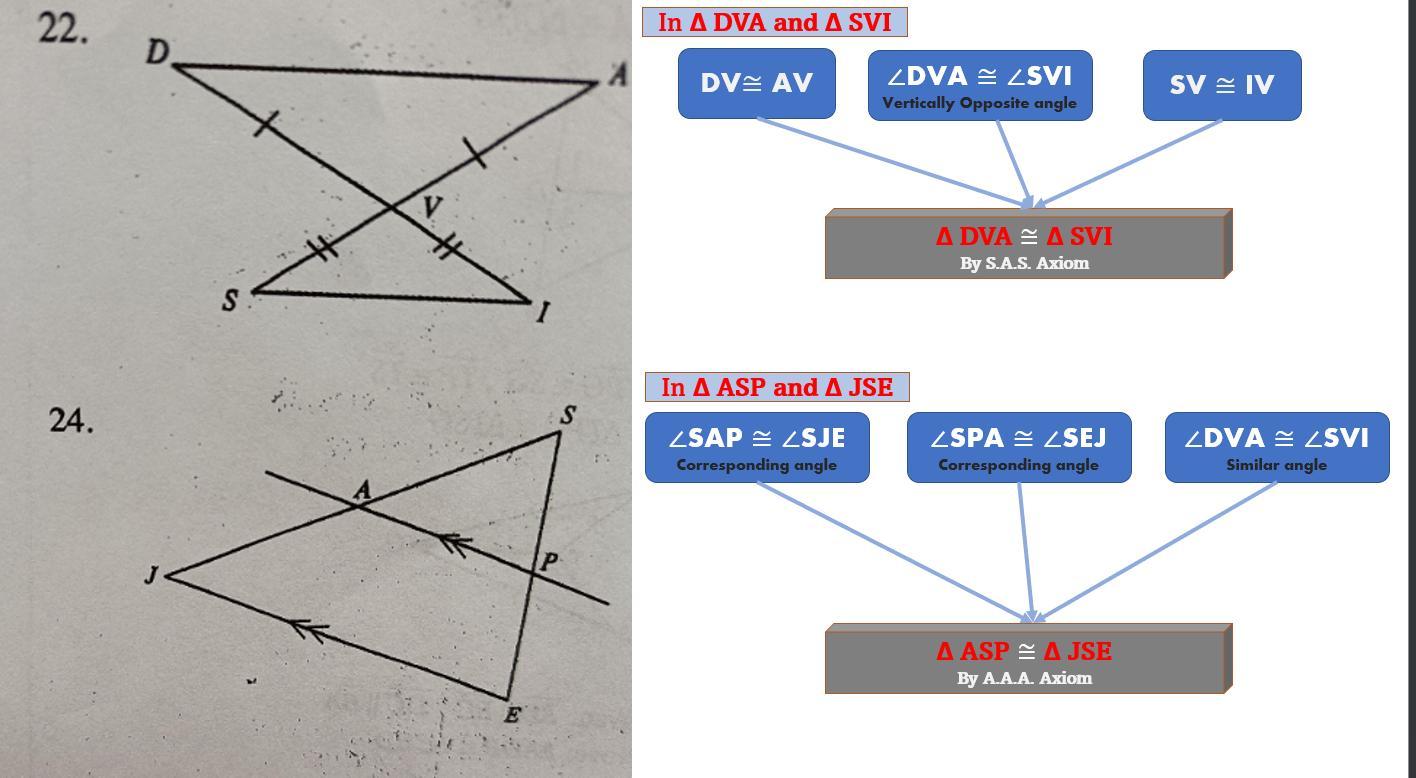
You stand a known distance from the base of the tree, measure the angle of elevation the top of the tree to be 15◦ , and then compute the height of the tree above eye level. Use the appropriate linear approximation to estimate the maximum possible error in your measurement of the angle (measuered in degrees) to be sure that your computation of the height has a relative error of at most ±p%. Give an exact answer, simplified as much as possible. Do not use a calculator. Assume p ∼ 0.
Answers
Answer:
The maximum possible error of in measurement of the angle is \(d\theta_1 =(14.36p)^o\)
Step-by-step explanation:
From the question we are told that
The angle of elevation is \(\theta_1 = 15 ^o = \frac{\pi}{12}\)
The height of the tree is h
The distance from the base is D
h is mathematically represented as
\(h = D tan \theta\) Note : this evaluated using SOHCAHTOA i,e
\(tan\theta = \frac{h}{D}\)
Generally for small angles the series approximation of \(tan \theta \ is\)
\(tan \theta = \theta + \frac{\theta ^3 }{3}\)
So given that \(\theta = 15 \ which \ is \ small\)
\(h = D (\theta + \frac{\theta^3}{3} )\)
\(dh = D (1 + \theta^2) d\theta\)
=> \(\frac{dh}{h} = \frac{1 + \theta ^2}{\theta + \frac{\theta^3}{3} } d \theta\)
Now from the question the relative error of height should be at most
\(\pm p\)%
=> \(\frac{dh}{h} = \pm p\)
=> \(\frac{1 + \theta ^2}{\theta + \frac{\theta^3}{3} } d \theta = \pm p\)
=> \(d\theta = \pm \frac{\theta + \frac{\theta^3}{3} }{1+ \theta ^2} * \ p\)
So for \(\theta_1\)
\(d\theta_1 = \pm \frac{\theta_1 + \frac{\theta^3_1 }{3} }{1+ \theta_1 ^2} * \ p\)
substituting values
\(d [\frac{\pi}{12} ] = \pm \frac{[\frac{\pi}{12} ] + \frac{[\frac{\pi}{12} ]^3 }{3} }{1+ [\frac{\pi}{12} ] ^2} * \ p\)
=> \(d\theta_1 = 0.25 p\)
Converting to degree
\(d\theta_1 = (0.25* 57.29) p\)
\(d\theta_1 =(14.36p)^o\)
Solve for e
7 = 15 - 2e
e equals....

Answers
Answer:
e=4
Step-by-step explanation:
Which equation results from adding the equations in this system?
-8x+8y = 8
3x-8y=-18
A: 5x=-10
B: 5x = 26
C: -5x = 26
D: -5x=-10
Answers
Answer:
D. - 5x = - 10Step-by-step explanation:
Given equations:
-8x+8y = 8 3x-8y=-18Add together:
-8x + 8y + 3x - 8y = 8 - 18- 5x = - 10Correct option is D
Step-by-step explanation:
The correct answer is letter D
Alyssa planted 2 rows of onions and 2 rows of turnips in a garden. Alyssa also
planted 2 a row of oak trees. In total, how many rows of vegetables
did Alyssa plant?
Answers
Answer:
7\(\frac{1}{2}\) rows
Step-by-step explanation:
just count the rows! (if she planted half a row then add it as half)
2\(\frac{1}{2}\) + 2\(\frac{1}{2}\) + 2\(\frac{1}{2}\) (you can split it up)
2 + 2 + 2 + \(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\)
6 + 1\(\frac{1}{2}\) = 7\(\frac{1}{2}\)
A.
B.
C.
D.
(I think it’s C)

Answers
Answer:
The answer would be C. I believe it is C. The force believes it is C. So put C.
please help i’m in need for it bruh

Answers
Answer:
70.68 inch is the volume of the rectangular prism
Step-by-step explanation:
V = w × h × l
V = 3.8 inch × 3 inch × 6.2 inch
V = 70.68 inch is the volume of the rectangular prism
To check:
70.68 ÷ 3.8 ÷ 6.2 = 3 inch (height)
70.68 ÷ 6.2 ÷ 3 = 3.8 inch (width)
70.68 ÷ 3.8 ÷ 3 = 6.2 inch (length)
What does 4! equal? Show the equation and what it equals
Answers
Answer:
4x3x2x1=24
Step-by-step explanation:
Please help!!! Banking

Answers
Answer:
deposit: 2
withdrawal: 3
transaction: 1
transfer: 4
Shelia walks to school 35% of the time. she rides the bus 24% of the time. what percentage will she be a car rider
Answers
Answer
Percentage of times that she goes to school by car = 41%
Step-by-step explanation
It is given that Shelia walks to school 35% of the time and rides the bus 24% of the time.
Given that she only has 3 alternative ways to get to school (walking, taking the bus or going to school by car), it means the remaining of the time, she rides a car.
(Percentage of times that she walks to school) + (Percentage of times that she takes the bus) + (Percentage of times that she goes to school by car) = 100%
35% + 24% + (Percentage of times that she goes to school by car) = 100%
59% + (Percentage of times that she goes to school by car) = 100%
Percentage of times that she goes to school by car = 100% - 59%
Percentage of times that she goes to school by car = 41%
Hope this Helps!!!
PLEASE HELP ME WITH THE ANSWER! THANK YOU!

Answers
f(x) = -2x^2+3x-6
how does the function open
Answers
20 POINTS
Part E
How many of the 320 million Americans would you predict wear glasses?
Answers
Help me answer this please

Answers
The area of the sector in terms of π is 35.6π inches squared.
The area of the sector is approximately 111.6 inches square
How to find the area of a sector?The area of sector of a circle is the amount of space enclosed within the boundary of the sector.
Therefore,
area of a sector = ∅ / 360 × πr²
where
r = radius∅ = central angleTherefore,
∅ = 200 degrees
r = 8 inches
area of the sector = 200 / 360 × 8²π
area of the sector = 200 / 360 × 64π
area of the sector =12800π/ 360
area of a sector = 35.6π inches squared
Let's find the area of the sector with π = 3.14
area of a sector = 35.6 × 3.14 = 111.6 inches square
learn more on sector here: https://brainly.com/question/22972014
#SPJ1
Write an expression to represent: 9 more than the quotient of 2 and x
Answers
Expression for given numerical is: 9+ (2/x)
Given that we have an word equation that is 9 more than the quotient of 2 and x
A quotient is a quantity produced by the division of two numbers.
Quotient of 2 and x is \(\frac{2}{x}\).
Nine(9) more than 2/x is = 9+ (2/x)
So we have an expression that is:
9+ (2/x)
learn more about quotient on brainly.com/question/16134410
#SPJ9
Solve the following equation and show your work: 13=-3(x-4)-8
Answers
Refer to photo attached below for further explanation:

need help asap please

Answers
It should be A. The distance between Jupiter and Earth is Greater than the distance between Mercury and Earth.
What is the value of y???? I NEED ANSWERS!!!! Please and thank you!!!

Answers
Answer:68
Step-by-step explanation:
angle sum property
56+y+y-12=180
44+2y=180
2y=180-44=136
y=136/2
=68
VERIFICATION :56+68+56
=180
Y =112°
remember the sum of angles in a triangle equals 180°
EDIT : previous answer is right, i messed up with the sign

The value of a piece of factory equipment was $20,000 when it was purchased. During each 1 -year period after the equipment was purchased, the value of the equipment at the end of the period was 10% less than its value at the beginning of the period. Which of the following statements identifies the most appropriate type of function to model this scenario and provides a reason to support its appropriateness?
A- A linear function is appropriate to model this scenario because, at the end of each year after purchase, the value of the equipment was $10 less than its value 1 year before.
B- A linear function is appropriate to model this scenario because, at the end of each year after purchase, the value of the equipment was $2,000 less than its value 1 year before.
C- An exponential function is appropriate to model this scenario because, at the end of each year after purchase, the value of the equipment was 0.1 times its value 1 year before.
D- An exponential function is appropriate to model this scenario because, at the end of each year after purchase, the value of the equipment was 0.9 times its value 1 year before.
Answers
The correct option for the function in this problem is:
D- An exponential function is appropriate to model this scenario because, at the end of each year after purchase, the value of the equipment was 0.9 times its value 1 year before.
What is an exponential function?A decaying exponential function is modeled by:
\(A(t) = A(0)(1 - r)^t\)
In which:
A(0) is the initial value.r is the decay rate, as a decimal.For this problem, during each 1 -year period after the equipment was purchased, the value of the equipment at the end of the period was 10% less than its value at the beginning of the period, hence r = 0.1 and the equation is:
\(A(t) = A(0)(1 - 0.1)^t\)
\(A(t) = A(0)(0.9)^t\)
Which means that option D is correct.
More can be learned about exponential functions at https://brainly.com/question/25537936
#SPJ1
i need help asap
than you!

Answers
The highest height for the daily rainfall were for 5 and 6 inches
How to Interpret Dot Plots?Dot plots are used to present data in the form of points or small circles. It is similar to a simplified histogram or bar graph in that the height of the bar made up of points represents the numerical value of each variable. Dot plots are used to represent small amounts of data.
From the given dot plot, we see the number of times for each height of rainfall.
Thus, we see that the highest number of times of rainfall height was from that of 5 and 6 inches.
Thus, we conclude those were the highest heights for the daily rainfall.
Read more about Dot plots at: https://brainly.com/question/24309209
#SPJ1
I need help ASAP with both

Answers
Answer:
No for the first one, yes for the second.
Step-by-step explanation:
Plug in the given numbers and see if it works.
15(1) + 3(4) = 10
15 + 12 = 10.
27 ≠ 10
Since 27 does not equal 10, we can already see that solution (1,4) will not work for these equations.
Now for the second problem, do the same thing.
10 = 1 + 9.
This is true, but remember to check the second equation just to be sure.
10 = 4(1) + 6
10 = 4 + 6
This is also true, so it (1,10) is a solution for the second system of equations.
please help me
this question is too much hard
I think that my brain stopped working

Answers
Please Help 2
Find (f/g)(x) if f(x)=x2+x+1 and g(x)=-x2-x-1

Answers
Answer:
-1
Step-by-step explanation:
Take -1 as common form the denominator then cancel out the whole term i.e x²-x-1 and -1 remains.
The point (–3, –5) is on the graph of a function. Which equation must be true regarding the function?
a. f(–3) = –5
b. f(–3, –5) = –8
c. f(–5) = –3
d. f(–5, –3) = –2
Answers
Answer:
a. f(–3) = –5
Step-by-step explanation:
On a graph, point (x, y) can be also represented as function f (x) = y
it means that if f(x) has input value as x then its output value will be y.
Now given that in the problem that
The point (–3, –5) lies on the graph
it means that for function input value of of -3 has the out put value of -5
which can also be written as
f(-3) = -5
Hence the correct choice is a. f(–3) = –5.